cgrap.f File Reference

Go to the source code of this file.

Functions/Subroutines
subroutine	cgrap (w, ptav, ntp, a, icon)
subroutine	cctrap (g, n, y, z)
subroutine	cccrap (g, n, sume, sump)
subroutine	cnprap (g, n, maxi, mini)
subroutine	ccmrap (w, g, n, maxi, mini, y, z, icon)
subroutine	cprap (ptav, pc, n)
subroutine	cprap0 (a, y)

Function/Subroutine Documentation

cccrap()

subroutine cccrap	(	type(ptcl), dimension(n)	g,
		integer	n,
		real*8	sume,
		real*8	sump
	)

Definition at line 58 of file cgrap.f.

References d0.

!                 check conservation
!      *******************************************************
       implicit none
!----       include '../../Zptcl.h'
#include  "Zptcl.h"
       integer n
       type(ptcl):: g(n)
       real*8 sume, sump
!
       integer i
       real*8 yr
!
        sume=0.d0
        sump=0.d0
        do   i=1, n
!               yr=g(i).fm.rap
                yr=g(i)%fm%p(4)
!               sume=sume+ cosh(yr)*g(i).fm.tm
!               sump=sump+ sinh(yr)*g(i).fm.tm
               sume=sume+ cosh(yr)*g(i)%fm%p(3)
               sump=sump+ sinh(yr)*g(i)%fm%p(3)
        enddo

ccmrap()

subroutine ccmrap	(	real*8	w,
		type(ptcl), dimension(n)	g,
		integer	n,
		integer	maxi,
		integer	mini,
		real*8	y,
		real*8	z,
		integer	icon
	)

Definition at line 118 of file cgrap.f.

References true.

Referenced by cgrap().

!        get coefficients y and z to modify rapidities so that
!        the total energy and pz should be conserved.
!
!
!       w: real*8. input.  available energy
!    g(n): /ptcl/  input.  g(i).fm.tm and g(i).fm.rap are used.
!       n: integer. input.  number of particles
!    maxi: integer. input. g(maxi).fm.rap is the max y
!    mini: integer. input. g(mini).fm.rap is the min y
!       y: real*8   output. coefficient in rap <- z + y* rap'
!                   where rap is the true rapidity which
!                   satisfy the energy-momentum conservation.
!       z: real*8   output. coefficient in rap <- z + y* rap'
!    icon: integer  output. 0--> o.k
!                           1--> n.g. retry
!  see. cpc. vol9. (1975). 297 by Jadach.
!
!          function to be solved for y is  symmetric around y=0
!          and has a form like  f(y)= c - y**2 ( c> 0)
!          (y > 0 is obtained as a solution)
!          in some stragne input, c becomes <0 with no solution
        implicit none
!----        include '../../Zptcl.h'
#include  "Zptcl.h"
        integer n, icon
        type(ptcl):: g(n)
!
        real*8 w, y, z
        integer maxi, mini
!
        real*8 eps1/0.0010d0/, w2, alw2, y1, epsx
        real*8 sump, summ, sumgp, sumgm, tmp
        real*8 expgyp, expgym, fy1, fy1p, dy, eps
        integer lp, i
!
         w2=w*w
         alw2=log(w2)
!         y1=log(w2/g(maxi).fm.tm/g(mini).fm.tm)
         y1=log(w2/g(maxi)%fm%p(3)/g(mini)%fm%p(3))
         epsx=eps1/sqrt(dble(n))
!
         lp=0
!         *** until loop*** 
         do while (.true.)
              lp=lp+1
              y = y1
              sump=0.
              summ=0.
              sumgp=0.
              sumgm=0.
              do   i=1, n
!                  tmp=g(i).fm.rap*y
                  tmp=g(i)%fm%p(4)*y
                  if(tmp .gt. 100.d0 .or. 
     *                  tmp .lt. -100.d0) then
!                        no solution case: c < 0
                      lp=100
                      goto 100
                  else

                      expgyp=exp(tmp)
                      expgym=1.d0/expgyp
!                      sump = sump+ g(i).fm.tm*expgyp
!                      summ = summ+ g(i).fm.tm*expgym
                      sump = sump+ g(i)%fm%p(3)*expgyp
                      summ = summ+ g(i)%fm%p(3)*expgym
                      sumgp = sumgp +
!     *                     g(i).fm.tm*g(i).fm.rap*expgyp
     *                     g(i)%fm%p(3)*g(i)%fm%p(4)*expgyp
                      sumgm=sumgm +
!     *                     g(i).fm.tm*g(i).fm.rap*expgym
     *                     g(i)%fm%p(3)*g(i)%fm%p(4)*expgym
                  endif
               enddo

              fy1=alw2 -log(sump*summ)
              fy1p= - sumgp/sump + sumgm/summ
              dy =fy1/fy1p
              y1=y- dy
              eps=dy/y1
              if(abs(eps) .lt. epsx .or. lp .gt. 10) goto 100
         enddo
  100    continue
         if(lp .gt. 10) then
             icon=1
         else
             icon=0
             y=y1
             z=log(w/sump)
         endif

Here is the caller graph for this function:

cctrap()

subroutine cctrap	(	type(ptcl), dimension(n)	g,
		integer	n,
		real*8	y,
		real*8	z
	)

Definition at line 39 of file cgrap.f.

Referenced by cgrap().

!           convert rapidity into true rapidity satisfing e-p
!           conservation.
!      *******************************************************
       implicit none
!----       include '../../Zptcl.h'
#include  "Zptcl.h"
       integer n
       type(ptcl):: g(n)
       real*8 y, z
!       
       integer i
!
          do   i=1, n
!               g(i).fm.rap = g(i).fm.rap*y + z
               g(i)%fm%p(4) = g(i)%fm%p(4)*y + z
          enddo

Here is the caller graph for this function:

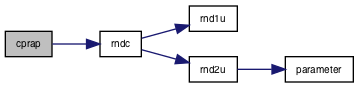
cgrap()

subroutine cgrap	(	real*8	w,
		real*8	ptav,
		integer	ntp,
		type(ptcl), dimension(ntp)	a,
		integer	icon
	)

Definition at line 3 of file cgrap.f.

References ccmrap(), cctrap(), cnprap(), and cprap().

Referenced by ccylps().

!          generation of rapidity for mass missing. mass
!      ****************************************************** 
       implicit none
!----       include  '../../Zptcl.h'
#include  "Zptcl.h"
       integer ntp, icon
       type(ptcl):: a(ntp)
       real*8  ptav, w   ! w is missing mass
!
       integer i, maxi, mini
       real*8 y, z
!                 sample proto-rapidity
         call cprap(ptav,  a,  ntp)
!                 normalize proto-rapidity to 0 to 1.
         call cnprap(a, ntp, maxi, mini)
!                 compute transverse mass.(note; save in pt pos.)
         do   i=1, ntp
!              a(i).fm.tm= sqrt(a(i).mass**2 + a(i).fm.p(3)**2)
              a(i)%fm%p(3)= sqrt(a(i)%mass**2 + a(i)%fm%p(3)**2)
         enddo
!              get coef. for y and z to modify rapidity
!              to conserve 4 momenta.
         call ccmrap(w, a, ntp, maxi, mini, y, z, icon)
         if(icon .eq. 0) then
!               convert to true rapidity satisfing 4 mom.
!               conservation.
             call cctrap(a, ntp, y, z)
!            ____________________________________________________
!                         check conservation
!            call cccrap(a,  ntp, sume, sump)
!            write(*,*) ' sume=',sume,' m  =',pj.mass,  ' sump=',sump
!            ~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
         endif

Here is the call graph for this function:

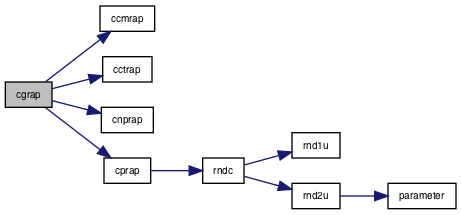
Here is the caller graph for this function:

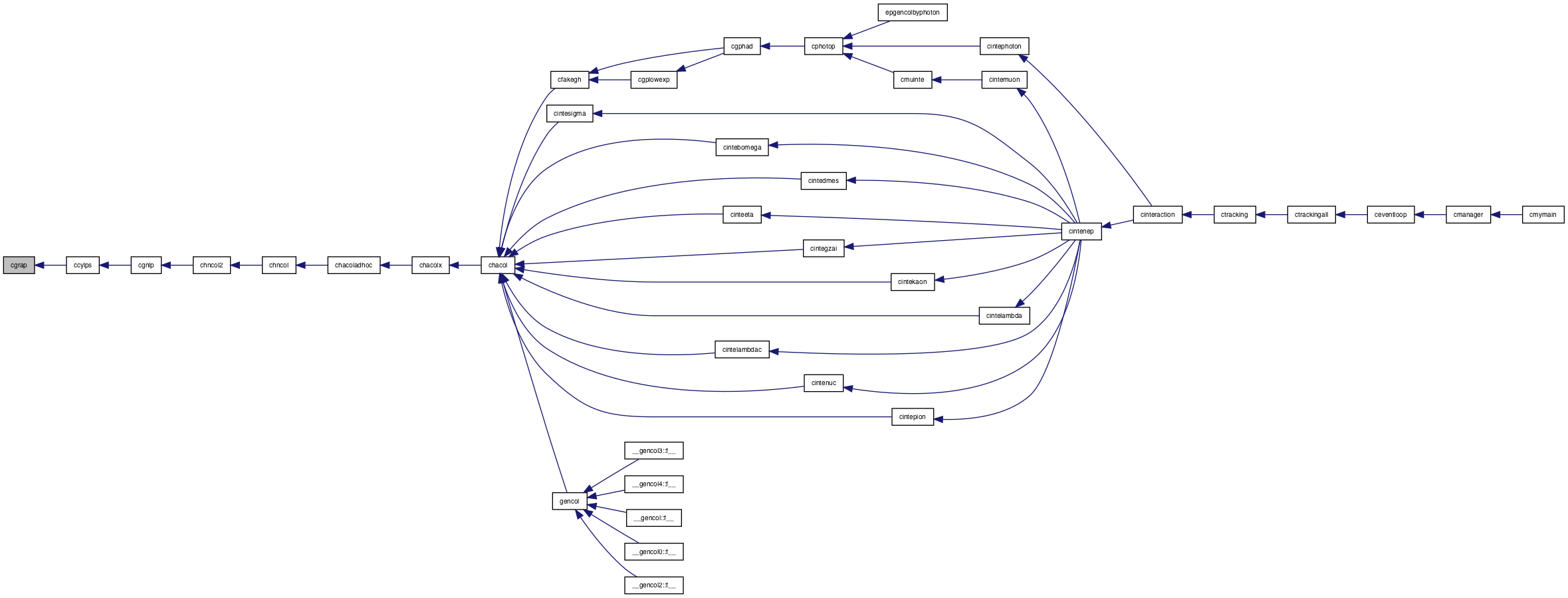
cnprap()

subroutine cnprap	(	type(ptcl), dimension(n)	g,
		integer	n,
		integer	maxi,
		integer	mini
	)

Definition at line 83 of file cgrap.f.

Referenced by cgrap().

!            normalize proto-rapidity in 0 to 1       
!      *********************************************
!       g(n): /ptcl/ Input.  g(i).fm.rap has y and
!             is normalized to have values in (0, 1.0).
!             the max value becomes 1.0 and the minimum one 0.0
!         n : integer Input. # of ptcls in g
!       maxi: integer. Output. g(maxi).fm.rap has  max y(=1)
!       mini: integer. Output. g(mini).fm.rap has  min y(=0)
!
       implicit none
!----       include '../../Zptcl.h'
#include  "Zptcl.h"
       integer n, maxi, mini
       type(ptcl):: g(n)
!
       integer i
       real*8 gmx, gmn
       maxi=1
       mini=1
       do   i=1, n
!          if(g(i).fm.rap .gt. g(maxi).fm.rap ) maxi=i
          if(g(i)%fm%p(4) .gt. g(maxi)%fm%p(4) ) maxi=i
!          if(g(i).fm.rap .lt. g(mini).fm.rap ) mini=i
          if(g(i)%fm%p(4) .lt. g(mini)%fm%p(4) ) mini=i
       enddo
!       gmx=g(maxi).fm.rap
!       gmn=g(mini).fm.rap
       gmx=g(maxi)%fm%p(4)
       gmn=g(mini)%fm%p(4)
       do   i=1, n
!          g(i).fm.rap = (g(i).fm.rap - gmn )/(gmx-gmn)
          g(i)%fm%p(4) = (g(i)%fm%p(4) - gmn )/(gmx-gmn)
       enddo

Here is the caller graph for this function:

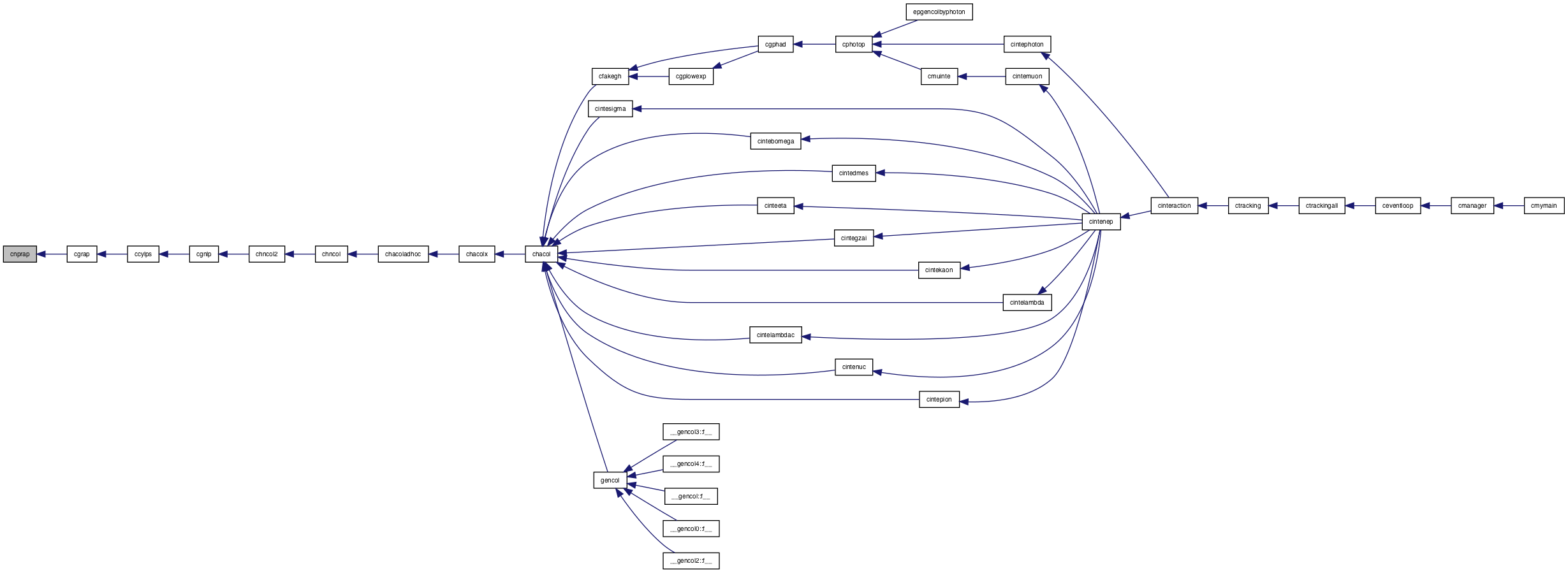
cprap()

subroutine cprap	(	real*8	ptav,
		type(ptcl), dimension(n)	pc,
		integer	n
	)

Definition at line 211 of file cgrap.f.

References d0, and rndc().

Referenced by cgrap().

!      *************************************
!            generate n proto-rapidities
!   ptav: real*8. input. avarage pt of this event
!  pc(n): /ptcl/  input/output. pc(i).fm.rap is created.
!      n: input. # of particles
!
!
!
       implicit none
!----       include  '../../Zptcl.h'
#include  "Zptcl.h"
       integer n
       type(ptcl):: pc(n)
       real*8 ptav
!
       integer i
       real*8 y, b, u
!
       do   i=1, n
!             b=(pc(i).fm.p(3)/ptav)**(-0.1) * 1.5   ! goot at 900 GeV

!             b=(pc(i).fm.p(3)/ptav)**(-0.2) * 1.5  !  goot at 900 GeV
!             b=(pc(i).fm.p(3)/0.3)**(-0.2) * 0.5   ! good at 200 GeV !
!              this  simple one is best.!!!
!             b= 1.  !  good at almost every where
             b = 1.0

!             call cprap0(b, y)

             call rndc(u)
             y =min(u*(ptav/pc(i)%fm%p(3))**0.40d0,  2.5d0)
             call rndc(u)
             if(u .lt.  0.5) then
                y = -y
             endif
                
!             pc(i).fm.rap = y
             pc(i)%fm%p(4) = y
       enddo

Here is the call graph for this function:

Here is the caller graph for this function:

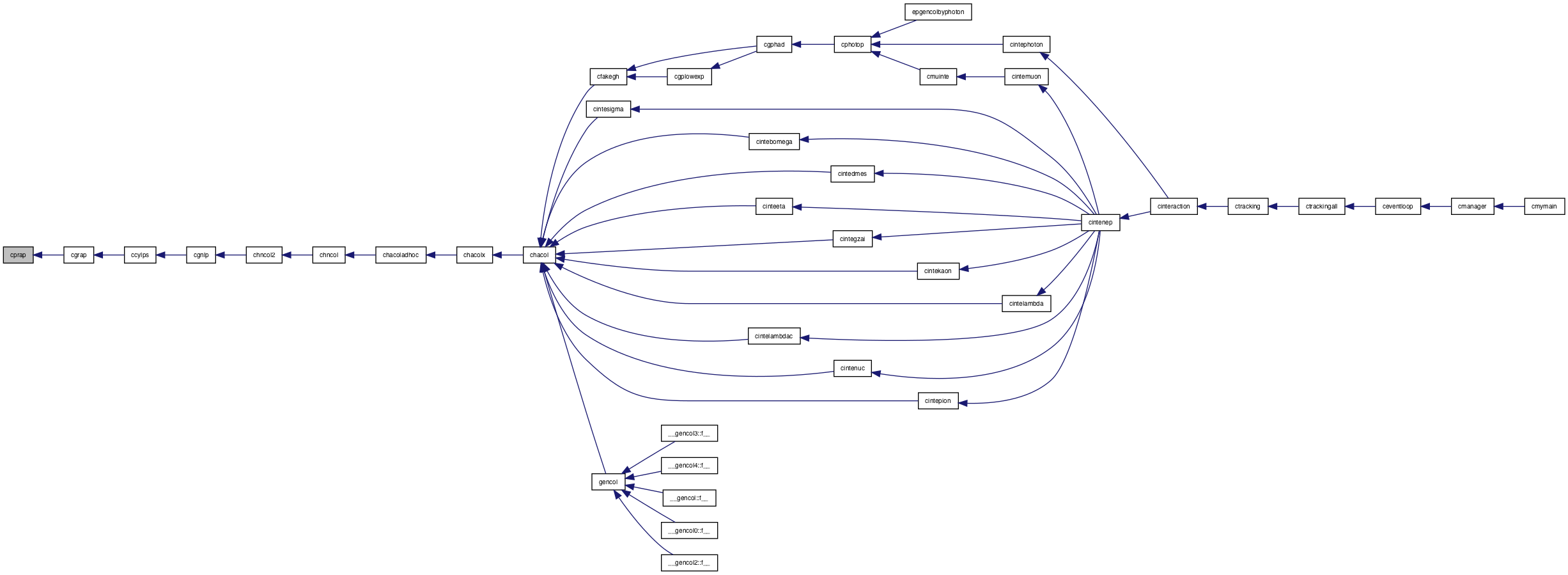
cprap0()

subroutine cprap0	(	real*8	a,
		real*8	y
	)

Definition at line 253 of file cgrap.f.

References d0, and rndc().

!         proto rapidity sampling
!     ***************************
       implicit none
       real*8 a, y
!
!             !
!           1 !*********!
!             !         !  *
!             !         !    *
!             !         !      *
!             !         !        *
!             !         !          *
!             0~~~~~~~~~1~~~~~~~~~~a+1
!
       real*8 s, u
          s=1.+a/2
          call rndc(u)
          if(u .lt. 1.d0/s) then
             call rndc(u)
             y=2*u-1.d0
          else
             call rndc(u)
             y=a*(1.d0 -sqrt(u)) + 1.d0
             call rndc(u)
             if(u .lt. 0.5d0) then
                y=-y
             endif
          endif

Here is the call graph for this function: